Cathodic protection monitoring is a most common practice for the assessment of effectiveness of corrosion protection.  However, metal loss due to corrosion is somehow evident even on pipes are deemed to be well protected based on standard monitoring and survey techniques.  The effectiveness of the the field survey techniques are further questioned for the structures within the common corridor with multiple functioning cathodic protection systems in place. Numeric simulation provides better opportunity to understand the pipe potential as current density along the structure. This, along with standard survey practice, renders a clear picture of the effectiveness of the cathodic protection.

Cathodic protection simulation is basically established a physical model by fundamental electrochemical theory. This physical model can be described as a mathematical model by establishing the governing differential equations with certain boundary conditions.  It is widely accepted that Laplace’s equation is applicable in the potential within field (Amaya, 2006). However, the boundary condition is far complicated than be taken as constant.  In practice, discrete coating failure leads to the different potential and current density distribution.  The metal polarization observes nonlinear  mathematical expression as well (A potential attenuation equation for cathodically polarized pipelines and risers, 2016). This leads to the numeric solution rather than analytical solution more feasible.

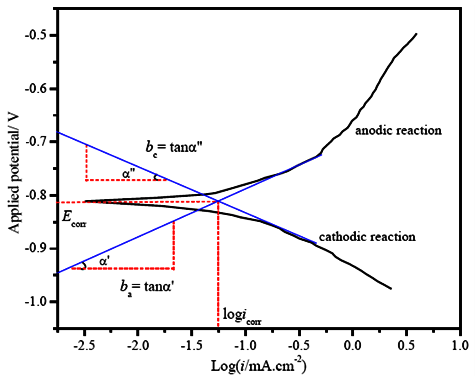
The polarisation of bare steel is compliant to Tefal equation as a boundary condition

The finite element method (FEM) and boundary element method (BEM (Pozrikidis, 2002)), as well as their hybrid (Muharemović & Letić, 2008), have been developed to solve these partial differential equations (PDE) models numerically.

Efforts have been made to simulate relevant features within the cathodic protection system, as well as interference to the system.

The features to be considered in the simulation:

• Complicate geometry of structure under the protection
• Coatings, including coating type, size and distribution of coating defects, coating thickness(Riemer & Orazem, 2006) (DeGiorgi & C.P.Hamilton, 1995)
• Anode current distribution
• Surrounding media resistitivities(Bortels & Deconinck, 2005)
• Pipe resistivities
• Auxiliary earthing

The interference to be considered in the simulations:

• Dynamic stray current e.g. caused by DC track
• Static stray current, e.g. nearby cathodic protected structure(Cui, Li, Yang, & Wang, 2016)
• Geomagnetic induced current, e.g. geo-storm
• HVAC induced AC interference (Leslie Bortels, 2006)

Some models are focusing on complicate geometry and current density  and potential distribution of the object (Diaz & Adey, 2005)

This simulation is especially useful to estimate the current demand in design stage. (P. Dmitruk, 1996) This is helpful in preventing the overprotection in sea water. (Tang, Zhang1, Liu1, & Li, 2009)

Bibliography

A potential attenuation equation for cathodically polarized pipelines and risers. (2016). Journals of Materials Research and Technology.

Amaya, K. (2006). Mathematical modeling for corrosion analysis. In Corrosion and Cathodic Protection Modelling and Simulation (pp. 1-12). WIT Press.

Bortels, L., & Deconinck, J. (2005). Numerical simulation of the cathodic protection of pipeline networks under various stray current interferences. In Corrosion and Cathodic Protection Modelling and Simulation (pp. 197-224). WIT Press.

Cui, G., Li, Z.-L., Yang, C., & Wang, M. (2016). The influence of DC stray current on pipeline corrosion. Petroleum Science, 135-145.

DeGiorgi, V., & C.P.Hamilton. (1995). Coating integrity effects on impressed current cathodic protection system parameters. In Transaction on Modelling and simulation. WIT Press.

Diaz, E., & Adey, R. (2005). Optimisation of cathodic protection systems. In Corrosion and Cathodic Protection Modelling and Simulation. WIT.

Leslie Bortels, J. D. (2006). A General Applicable Model for AC Predictive and Mitigation Techniques for Pipeline Networks Influenced by HV Power Lines. IEEE TRANSACTIONS ON POWER DELIVERY, 21, 210-17.

Muharemović, A., & Letić, H. Z. (2008). Modelling of protective potential distribution in a cathodic protection system using a coupled BEM/FEM method. In Boundary Elements and Other Mesh Reduction Methods (pp. 105-113). WIT Press.

Dmitruk, R. M. (1996). Estimation of cover resistance and protection electric current of a pipeline by means of the Boundary Element Method. In Transactions on Modelling and Simulation. WIT Press.

Pozrikidis, C. (2002). A practical guide to boundary element methods with the software library BEMLIB. Chapman & Hall/CRC.

Riemer, D., & Orazem, M. (2006). Modeling coating flaws with non-linear polarization curves for long pipelines. In Corrosion and Cathodic Protection Modelling and Simulation. WIT.

Tang, X., Zhang1, Y., Liu1, M., & Li, Y. (2009). Boundary Element Method (BEM) Analysis for Galvanic Corrosion of Hot Dip Galvanized Steel Immersed in Seawater. Journal of Materials Science and Technology, 194-198.